REVISION NOTES
IGCSE Edexcel Further Pure Mathematics
1.3 Identities and Inequalities
1.3.1 Simple algebraic division
1.3.2 The factor and remainder theorems
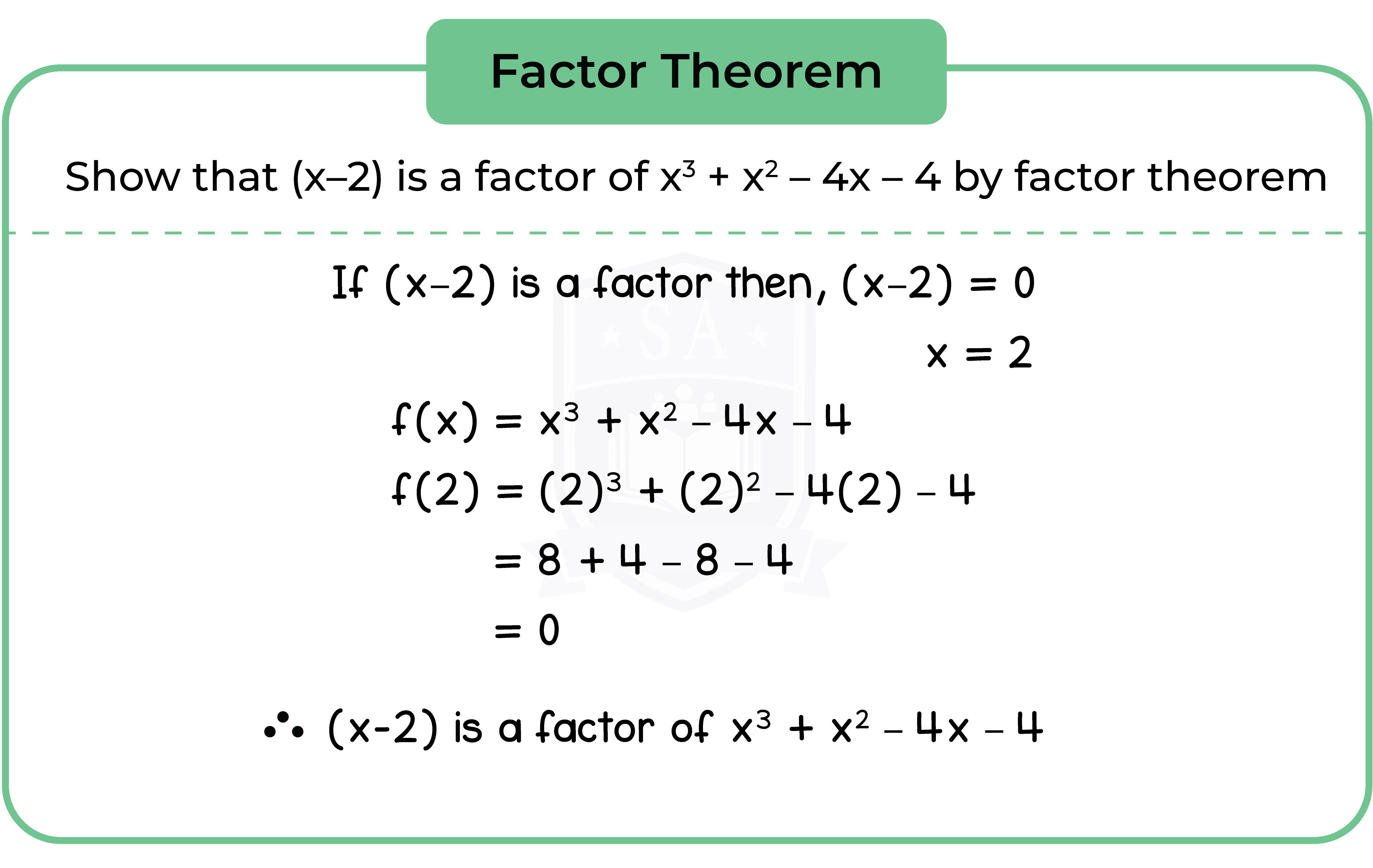
Part 1: Factor Theorem
If f(x) is a polynomial and f(p) = 0, the (x – p) is a factor of f(x).
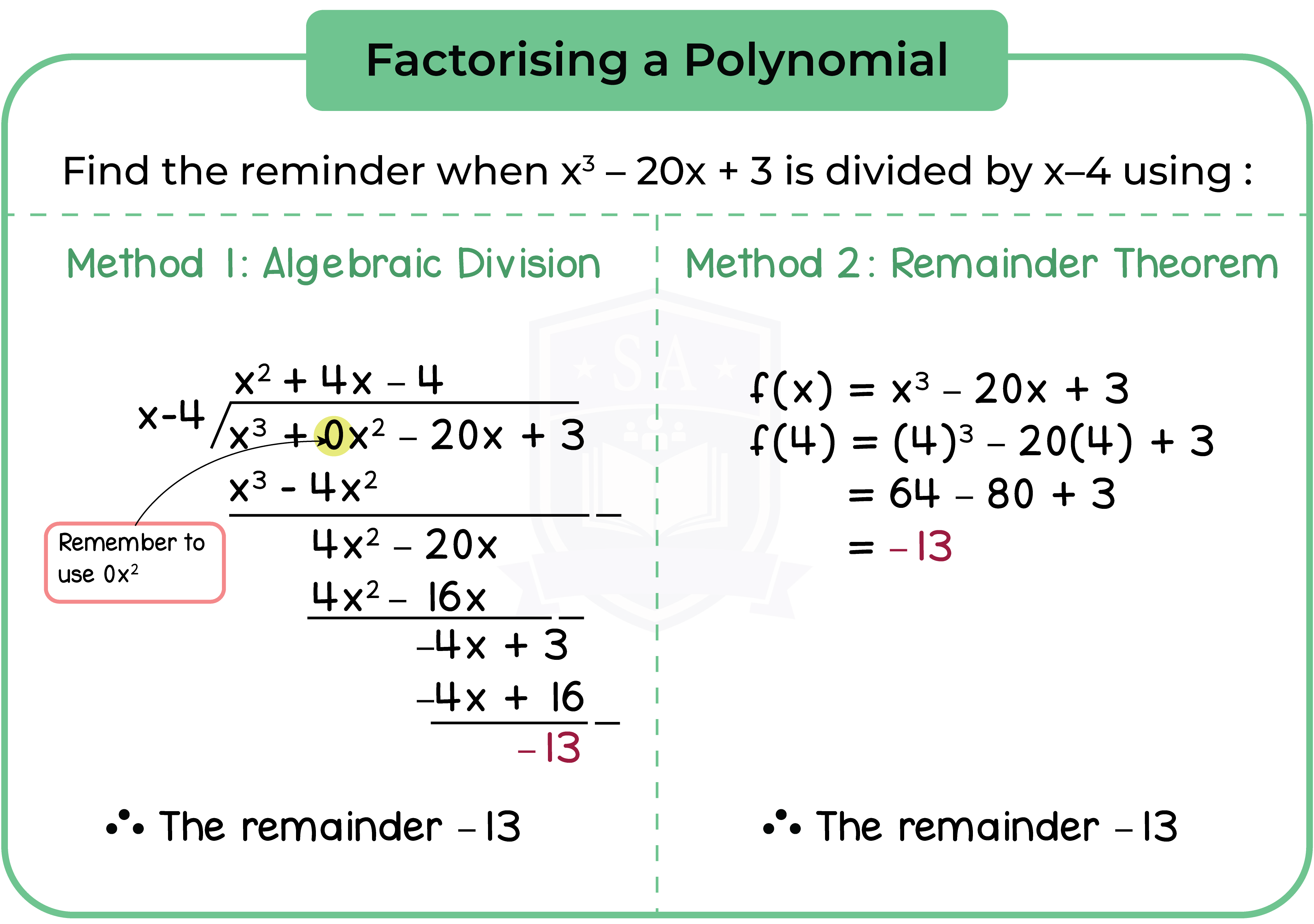
Part 2: Remainder Theorem
If a polynomial f(x) is divided by (ax – b), the remainder is f(b/a).
1.3.3 Simple inequalities, linear and quadratic
If two sides of an equation are not equal, use inequalities.
- > means more than
- < means less than
- ≤ means more than equal to
- ≥ means less than equal to
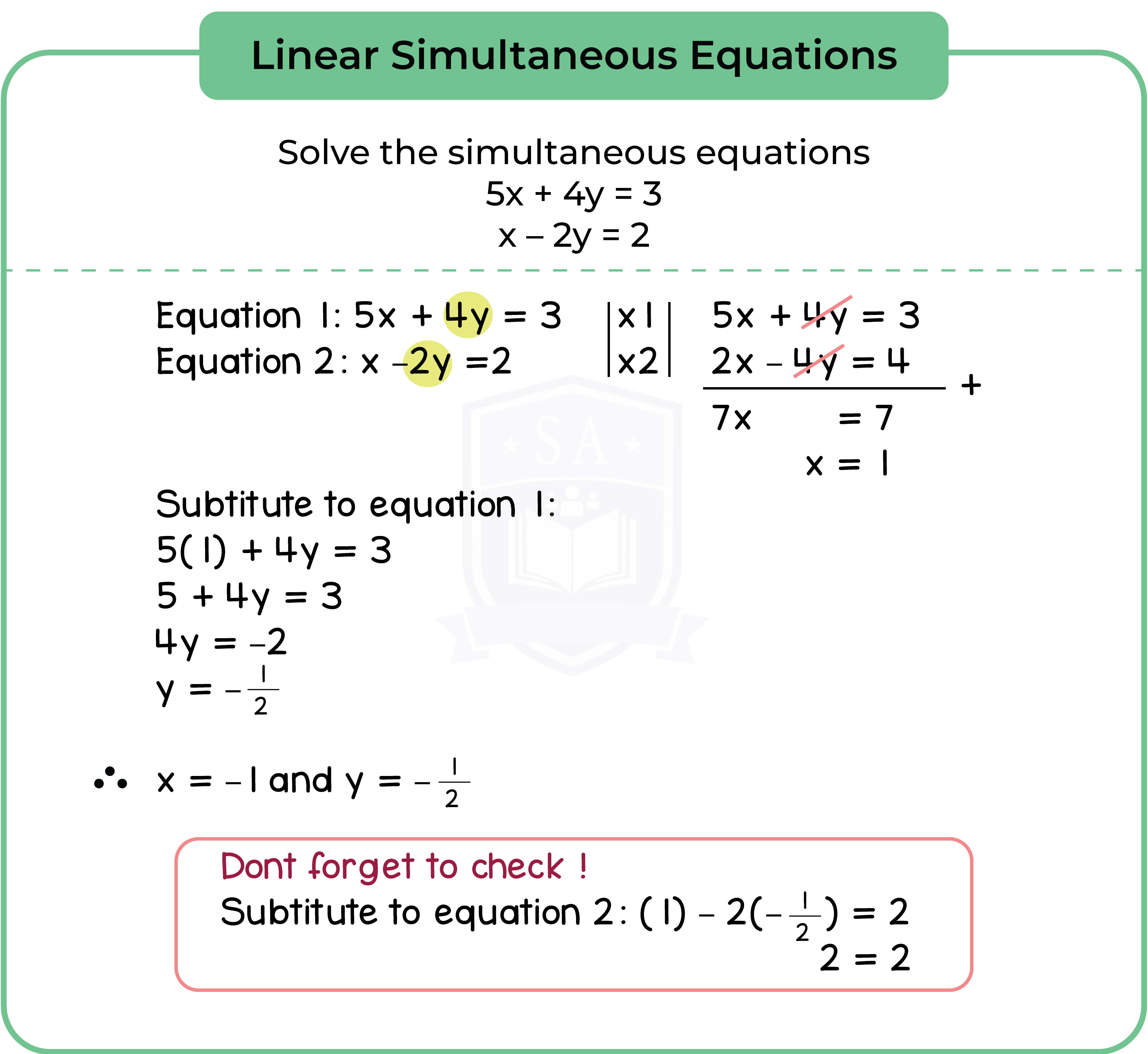
Type 1: Linear Inequalities
Solving linear inequalities is just like solving linear equations.
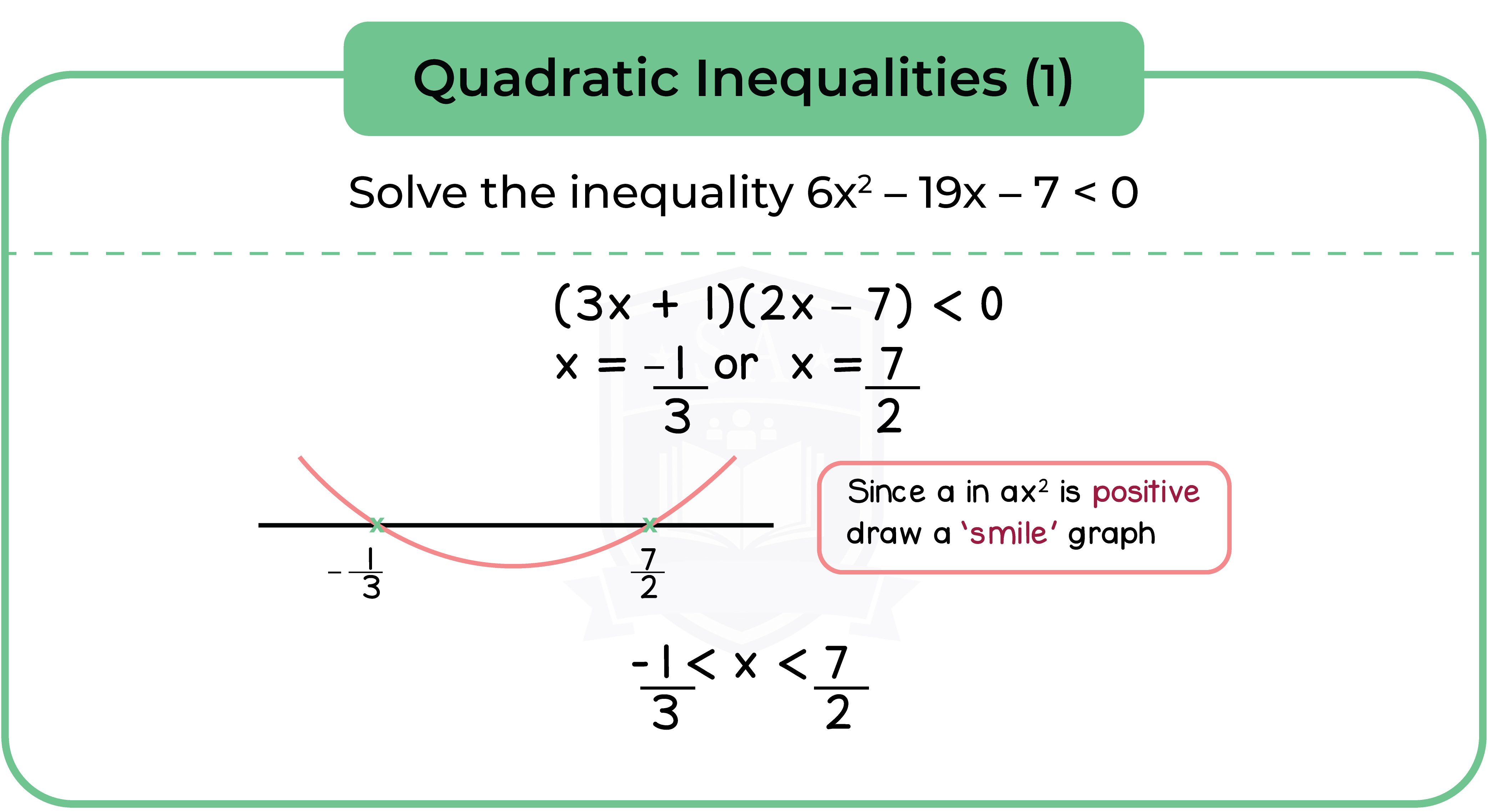
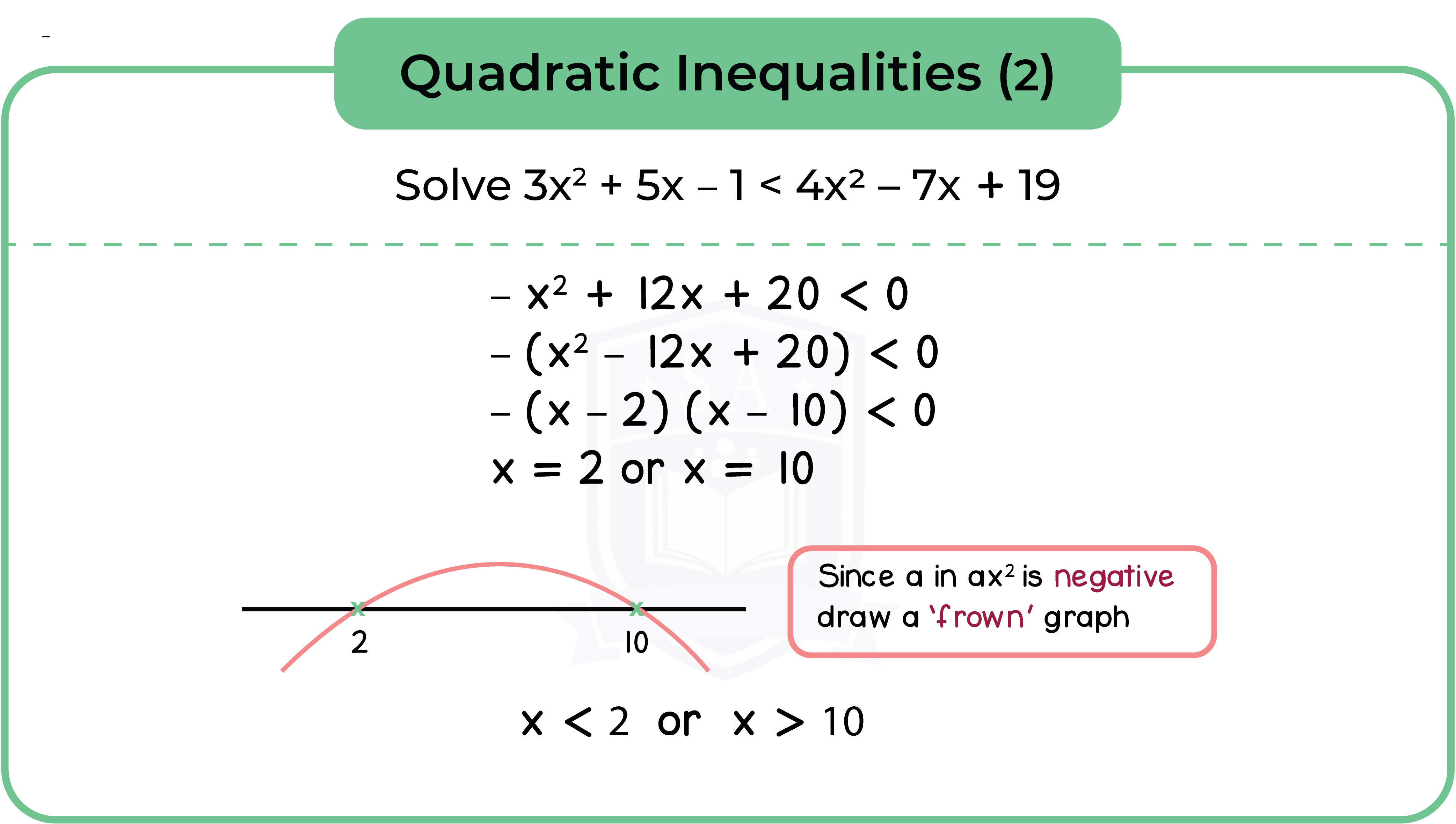
Type 2: Quadratic Inequalities
Step 1: Rearrange the quadratic equation into f(x) > 0 or f(x) < 0
Step 2: Factorise and solve the quadratic equation
Step 3: Sketch a quadratic graph using a number line
Step 4: Solve the quadratic inequalities
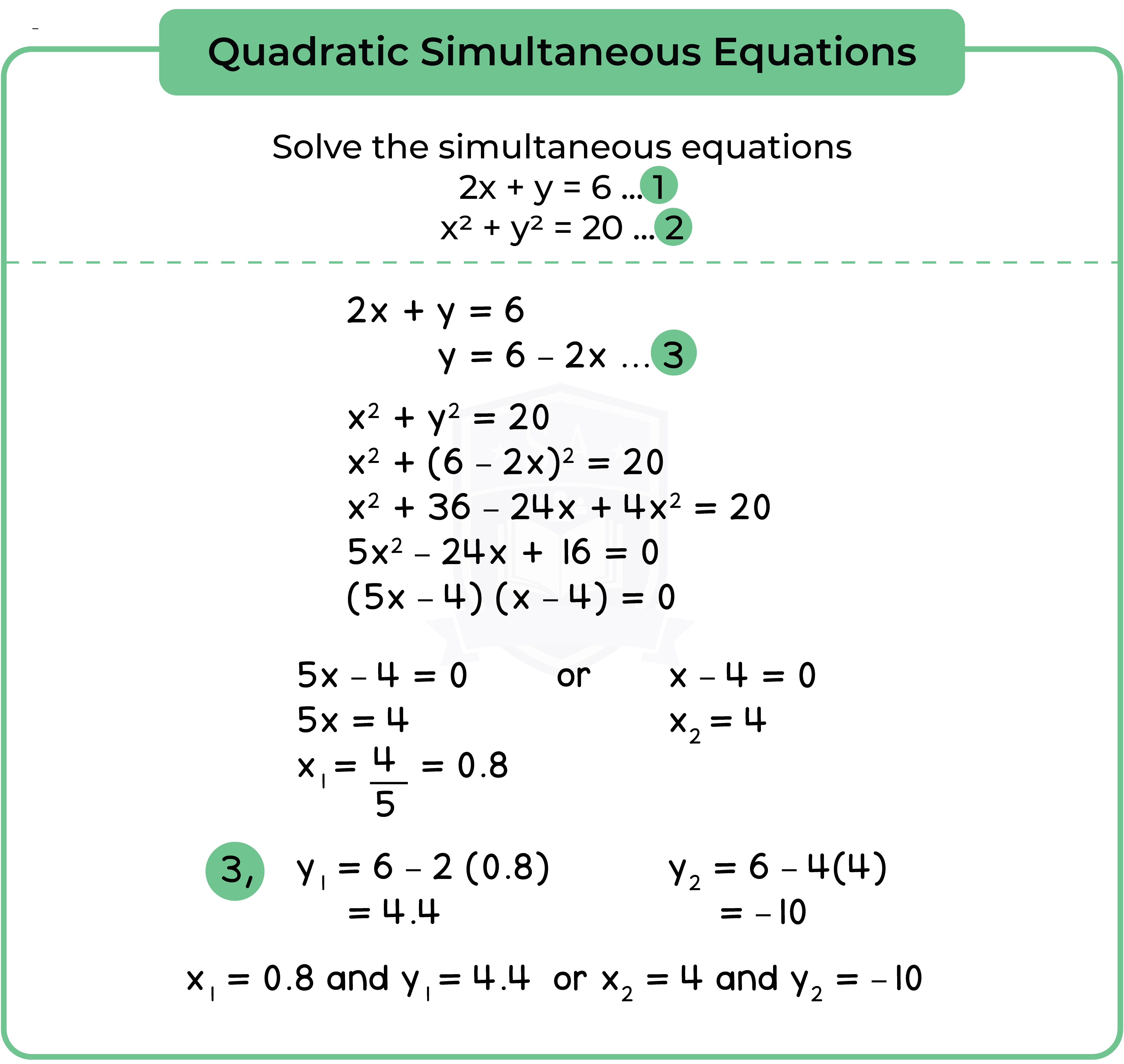
1.3.4 The graphical representation of linear inequalities in two variables
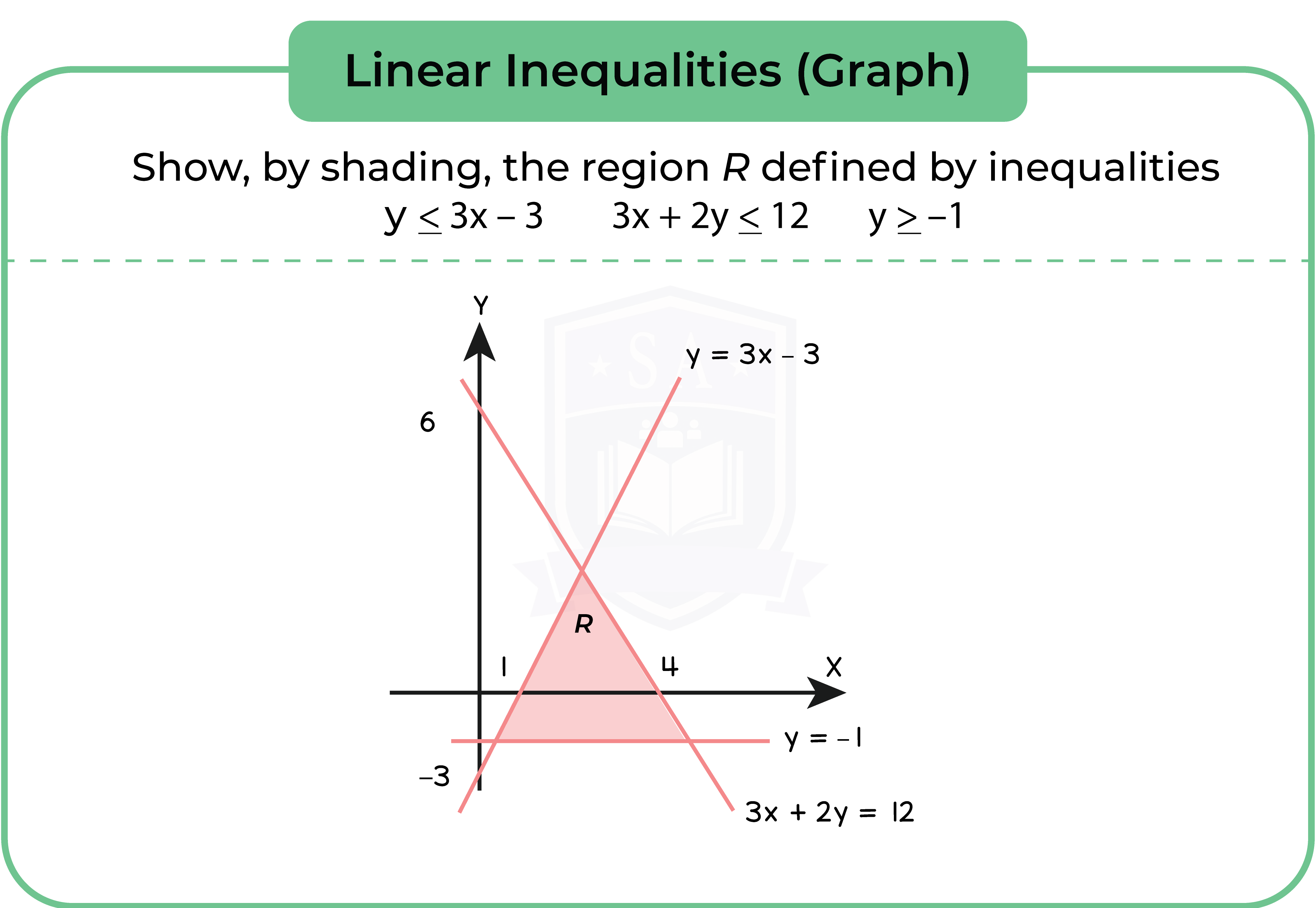
Type 1: Linear Inequalities (Graph)
Step 1: Draw the line for each equation
Step 2: Use a coordinate to determine whether the the region is true or not true
Step 3: Shade the region that is true
Step 4: Label the region R
